The "Quartic Formula"
The "Quartic Formula"
Our objective is to find two roots of the quartic equation
The other two roots (real or complex) can then be found by polynomial division and the quadratic formula. The solution proceeds in two steps. First, the quartic equation is "depressed"; then one reduces the problem to solving a related cubic equation.
We apply the substitution
to the quartic equation (1) to obtain:
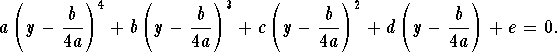
Multiplying out and simplifying, we obtain the "depressed" quartic
Let's try this for the example
Our substitution will be x=y-2; expanding and simplifying, we obtain the depressed quartic equation
We are left with solving a depressed quartic equation of the form
We will first move the y-term to the other side.
Next, we "complete the square" on the left side, but we want to end up with
. To accomplish that, we add the term
on both sides:
Simplifying the left side results in
Now here comes the crucial trick: Using a new unknown z, we want to change the left side of (5) to
which means we have to add 
to both sides of (5) to obtain:
What a mess! Let's see what we get for our concrete example Equation (2):
"Completing the square" in the fashion indicated above yields:
Since
adding the term 
to both sides of (7) results in
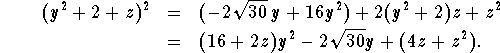
The left side is a "perfect square". The next step will be to determine z so that the right side looks like a perfect square as well.
Note that the right side is a quadratic polynomial in y. Ending up with a perfect square is just another way of saying that this polynomial has a repeated factor. A quadratic polynomial has a repeated factor if its discriminant is 0. Let's compute the discriminant in our example:
This polynomial is called the resolvent cubic polynomial for the quartic equation.
We can find a real root of the resolvent cubic; in this case, the Rational Zero Test reveals that z=-5 is such a root.
Where are we? If z=-5, then
is supposed to be a perfect square. Let's check that.
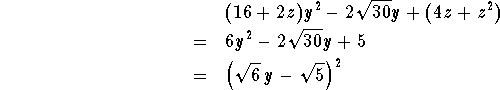
Bingo! By now, Equation (7) looks like this:
We're nearly done. Let's take square roots on both sides:
We discard one of the two choices and end up with
This is a quadratic equation! Its roots are
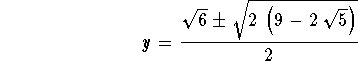
Using the substitution x=y-2, we see that our original equation
has two roots of the form
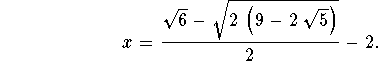
The other two roots can be found by polynomial long division, and application of the quadratic formula.
Let me finish by convincing you that making the right side a perfect square always amounts to solving a cubic equation: In the last general step (Equation (6)), we had written our depressed quartic equation as
Writing the right side neatly as a polynomial in y, we obtain
The discriminant of the right side is therefore
a cubic polynomial in z.
Thus the procedure outlined will work in general. One word of caution: A quartic equation may have four complex roots; so you should expect complex numbers to play a much bigger role in general than in my concrete example. In my discussion of the general case, I have, for example, tacitly assumed that C is positive.
Check that z=3 is a root of the resolvent cubic for the equation, then find all roots of the quartic equation.
S.O.S MATHematics home page 
Mon Jun 30 13:10:51 MDT 1997