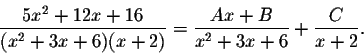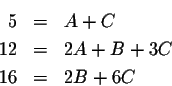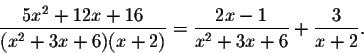The Method of Partial Fractions

2. The polynomial x²+3x+6 is irreducible, thus the setup looks like this:

$\begin{displaymath}\frac{5x^2+12x+16}{(x^2+3x+6)(x+2)}=\frac{Ax+B}{x^2+3x+6}+\frac{C}{x+2}.\end{displaymath}$

Multiplying by the least common denominator and "sorting" results in

5x²+12x+16=(2A+C)x²+(A+B+3C)x+(2B+6C).

By comparing the polynomial coefficients on both sides we obtain the requirements

$\begin{eqnarray*}5&=&A+C\\ 12&=&2A+B+3C\\ 16&=&2B+6C \end{eqnarray*}$

The coefficients turn out to be A=2, B=-1 and C=3, so

$\begin{displaymath}\frac{5x^2+12x+16}{(x^2+3x+6)(x+2)}=\frac{2x-1}{x^2+3x+6}+\frac{3}{x+2}.\end{displaymath}$

Helmut Knaust
Sat Oct 24 13:54:22 MDT 1998