EQUATIONS CONTAINING VARIABLES UNDER ONE OR MORE RADICALS

Note:

• In order to solve for x, you must isolate x.

• In order to isolate x, you must remove it from under the radical.

• If there is just one radical in the equation, isolate the radical.

• Then raise both sides of the equation to a power equal to the index of the radical.

• With these types of equations, sometimes there are extraneous solutions; therefore, you must check your answers.

• If the index of the radical is even, many times there will be a restriction on the values of x.

Problem 2.3e:

Answer: x=3.46939

Solution:

First make a note of the fact that you cannot take the square root of a negative number. Therefore, .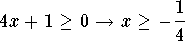

Subtract 5 from both sides of the equation so that the radical term is isolated.

Square both sides of the equation and simplify:

Subtract 100x+25 from both sides of the equation.

Solve using factoring:

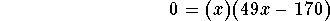
or

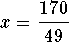
The answers are

Check the solution x=0 by substituting 0 for x in the original equation. If after the substitution, the left side of the original equation equals the right side of the original equation, 0 is a solution.

• Left Side:

• Right Side: (0)=0

Since the left side of the original equation does not equal the right side of the original equation after 0 was substituted for x, x=0 is not a valid solution.

Check the solution x=3.46939 by substituting 3.46939 for x in the original equation. If after the substitution, the left side of the original equation equals the right side of the original equation, 3.46939 is a solution.

• Left Side:

• Right Side: (3.46939) = 24.28572

Since the left side of the original equation not equals the right side of the original equation after 3.46939 was substituted for x, then x=3.46939 is a valid solution.

You can check the answer by graphing the equation:

The graph represents the right side of the original equation minus the left side of the original equation. You can see that there is one x-intercept, at x=3.46939. This means that there is one solution x=3.45939.

If you would like to go back to the problem page, click on Problem.

If you would like to go back to the equation table of contents, click on Contents.

[Algebra] [Trigonometry] [Geometry] [Differential Equations] [Calculus] [Complex Variables] [Matrix Algebra]

S.O.S. MATHemathics home page