SOLVING EQUATIONS CONTAINING ABSOLUTE VALUE(S)

Note:

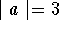• if and only if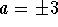

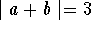• if and only if a + b = 3 or a + b = -3

• Step 1: Isolate the absolute value expression.

• Step 2: Set the quantity inside the absolute value notation equal to + and - the quantity on the other side of the equation.

• Step 3: Solve for the unknown in both equations.

• Step 4: Check your answer analytically or graphically.
  

Solve for x in the following equation.

Problem 3.2c :

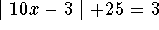
Step1: Isolate the absolute value expression by subtracting 25 from both sides of the equation.

Step 2: Since the absolute value can never be negative, there is no solution. You can also check your answer by graphing

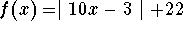
(the left side of the original equation minus the right side of the original equation).

You will note that there are no x-intercepts on the graph. Therefore, there are not solutions to this problem.

If you would like to go back to the problem page, click on Problem.

If you would like to go back to the equation table of contents, click on Contents.

[Algebra] [Trigonometry] [Geometry] [Differential Equations] [Calculus] [Complex Variables] [Matrix Algebra]

S.O.S MATHematics home page

Copyright © 1999-2004 MathMedics, LLC. All rights reserved.
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA