 SOLVING QUADRATIC EQUATIONS
SOLVING QUADRATIC EQUATIONS SOLVING QUADRATIC EQUATIONS
SOLVING QUADRATIC EQUATIONS

Note:
 Factoring
Factoring
 Completing the Square
Completing the Square
 Quadratic Formula
Quadratic Formula
 Graphing
Graphing
Solve for x in the following equation.
Problem 4.1D 
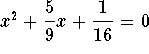
Answer: 
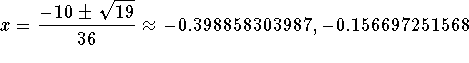
Solution:  The equation is already equal to zero.
The equation is already equal to zero.
Method 1:  Factoring
Factoring
The equation cannot be easily factored.
Method 2:  Completing the square
Completing the square
Subtract ![]() from both sides of the equation.
from both sides of the equation.
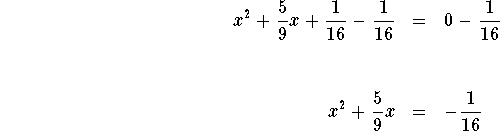
Add 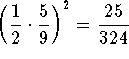 to both sides of the equation.
to both sides of the equation.
![]()
Factor the left side and simplify the right side.
![]()
Take the square root of both sides of the equation,
![]()
Subtract ![]() from both sides of the equation.
from both sides of the equation.
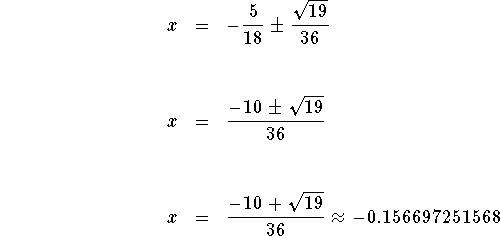
![]()
Method 3:  Quadratic Formula
Quadratic Formula
The quadratic formula is 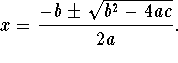
In the equation 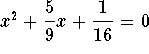 , a is the
coefficient of the
, a is the
coefficient of the ![]() term, b is the coefficient of the x
term, and c is the constant. Simply insert 51 for a,
term, b is the coefficient of the x
term, and c is the constant. Simply insert 51 for a, ![]() for b, and
for b, and ![]() for c in the
quadratic formula and simplify.
for c in the
quadratic formula and simplify.
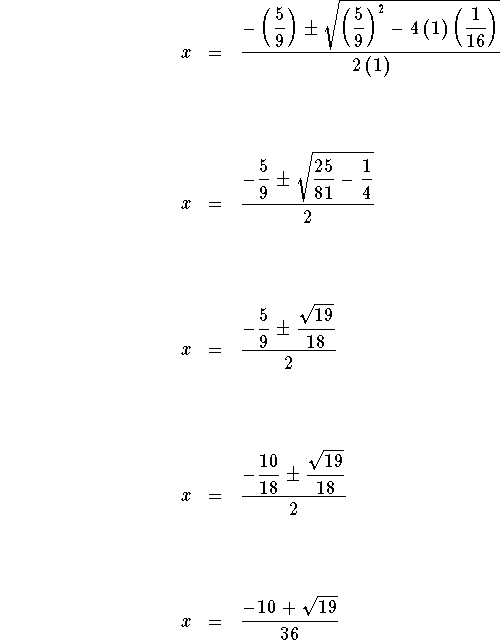
![]()
Method 4:  Graphing
Graphing
Graph y= the left side of the equation or
![]()
and graph y= the right side of the equation or y=0.The graph of y=0 is nothing more than the x-axis. So what you will be looking for is where the graph of
![]()
crosses the x-axis. Another way of saying this is that the x-intercepts are
the solutions to this equation.
The x-intercepts are -0.398858303987 and ![]() The answers are -0.398858303987 and
The answers are -0.398858303987 and ![]()
Check these answers in the original equation.
Check the answer x=-0.398858303987 by substituting -0.398858303987 in the original equation for x. If the left side of the equation equals the right side of the equation after the substitution, you have found the correct answer.

Check the solution x=-0.156697251568 by substituting -0.156697251568 in
the original equation for x. If the left side of the equation equals the
right side of the equation after the substitution, you have found the
correct answer.
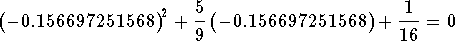
The solutions to the equation 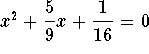 are
are
 and
and 
Comment:  You can use the solutions to factor the original equation.
You can use the solutions to factor the original equation.
For example, since 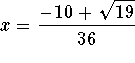 , then
, then 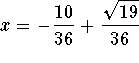 , and
, and 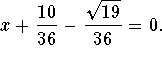
Since 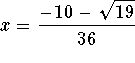 , then
, then 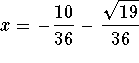 , and
, and 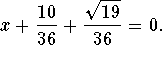
Since the product
![]()
and 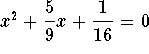 , then we can say that
, then we can say that
![]()
This means that
![]()
are factors of
![]()

