Note:
For an in-depth review on fractions, click on Fractions.
Solve for x in the following equation.
Problem 5.1b:
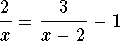
Answer:x = -1, 4
Solution:
Recall that you cannot divide by zero. Therefore, the first fraction is
valid if , 
and the second fraction is valid if 
If either 0 or 2 turn out to be solutions, you must
discard them as extraneous solutions.
The least least common multiple x(x-2) (the smallest expression that all the
denominators will divide into evenly) is 
. Multiply
both sides of the equation by the least common multiple
which is equivalent to
which can be rewritten as
which can be rewritten again as
which can be rewritten yet again as
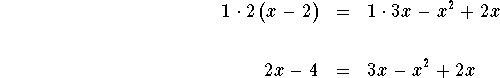
The answers are 
and 
Check this answers in the original equation.
Check the solution x=-1 by substituting -1 in the original equation for
x. If the left side of the equation equals the right side of the equation
after the substitution, you have found the correct answer.
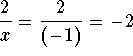
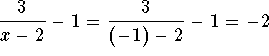
Since the left side of the original equation is equal to the right side of
the original equation after we substitute the value -1 for x, then x=-1
is a solution.
Check the solution x=4 by substituting 4 in the original equation for x.
If the left side of the equation equals the right side of the equation after
the substitution, you have found the correct answer.
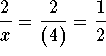
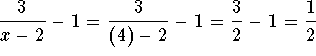
You can also check your answer by graphing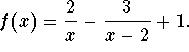 
(formed by subtracting the right side of the original
equation from the left side). Look to see where the graph crosses the
x-axis; that will be the real solution. Note that the graph crosses the
x-axis at -1 and 4. This means that the real solutions is -1and 4.
If you would like to go back to the problem page, click on Problem
If you would like to go back to the equation table of contents, click on
Contents
