Note:
If you would like an in-depth review of fractions, click on fractions
Solve for x in the following equation.
Problem 5.2g:
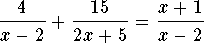
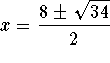are the exact answers and 
1.08452405258 are the approximate answer.
Comment on answers: You may wonder why we give you the answers in two forms:
exact and approximate. There is a reason. Students seem perplexed when they
think they have worked a problem correctly and yet, their exact answers
differ from the exact answers in the book. The student is not
necessarily wrong. Depending on the method chosen to work the problem,
exact answers have a different look. How do you know whether your exact
answer is equivalent to a different looking exact answer in the book?
Simplify both. If both exact answers are correct, they will both simplify to
the same approximate answer.
Next time your answer differs from the answer in the book, simplify both. If
the approximate answers are the same, you are correct. If not, go back to
the drawing board and try to find your mistake.
Solution:
Recall that you cannot divide by zero. Therefore, the first fraction is
valid if , 
the second fraction is valid if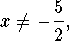 
and the third fraction is valid is 
.If either 
or 
turn out to
be the solutions, you must discard them as extraneous solutions.
Multiply both sides by the least common multiple 
(the smallest number that all the denominators
will divide into evenly). This step will eliminate all the
denominators.
which is equivalent to
which can be rewritten as
which can be rewritten as
which can be simplified to
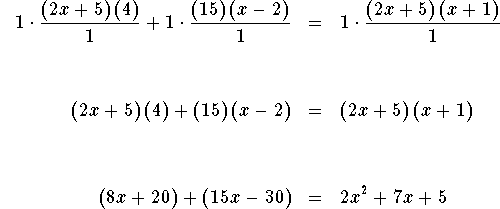
Solve for x using the quadratic formula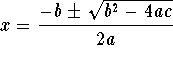 
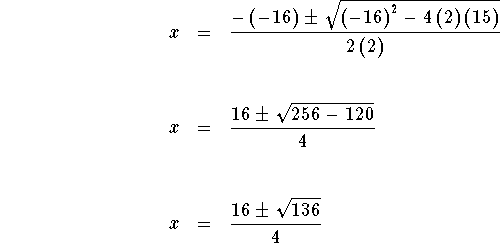
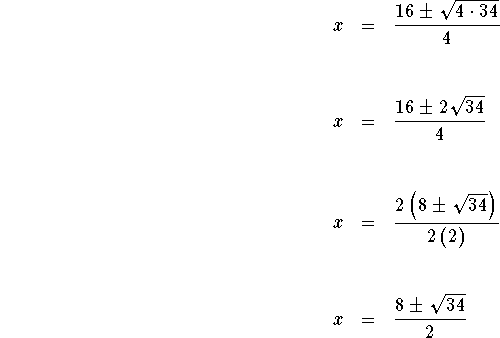
The answers are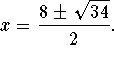 
However, this may or may not
be the answer. You must check the solution with the original equation.
Check the solution 
by substituting 6.91547594742
in the original equation for x. If the left side of the equation equals the
right side of the equation after the substitution, you have found the
correct answer.
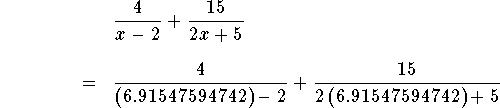
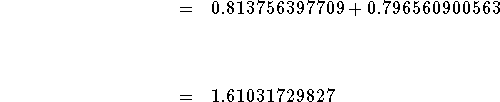
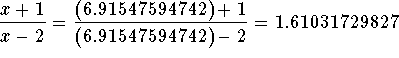
Check the solution 
by substituting 1.08452405258
in the original equation for x. If the left side of the equation equals the
right side of the equation after the substitution, you have found the
correct answer.
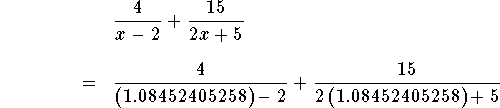
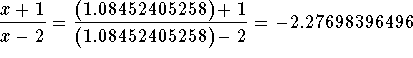
You can also check your answer by graphing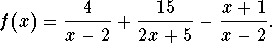 
(formed by subtracting the right side of
the original equation from the left side). Look to see where the graph
crosses the x-axis; that will be the real solution. Note that the graph
crosses the x-axis at two places: 
.
We have verified the solution two ways.
If you would like to review the solution to problem 5.2h, click on
Problem
If you would like to return to the beginning of this section, click on
Again
If you would like to go back to the equation table of contents, click on
Contents
This site was built to accommodate the needs of students. The topics and
problems are what students ask for. We ask students to help in the editing
so that future viewers will access a cleaner site. If you feel that some of
the material in this section is ambiguous or needs more clarification,
please let us know by e-mail.
