Using the Definition to Compute the Derivative

We have seen in the previous page how the derivative is defined: For a function f(x), its derivative at x=a is defined by

$$f'(a) = \lim_{x \rightarrow a} \frac{f(x) - f(a)}{x-a} = \lim_{h \rightarrow 0} \frac{f(a + h) - f(a)}{h}\cdot$$

Let us give some examples.

Example 1. Let us start with the function f(x) = x². We have

$$\frac{f(a + h) - f(a)}{h} = \frac{(a + h)^2 - a^2}{h} = \frac{2 a h + h^2}{h} = 2a + h.$$

So

$$\lim_{h \rightarrow 0} \frac{f(a + h) - f(a)}{h} = 2 a.$$

which means f '(a) = 2a.

What about the derivative of f(x) = xⁿ. Similar calculations, using the binomial expansion for (x+y)ⁿ (Pascal's Triangle), yield

$$f'(a) = n a^{n-1}\cdot$$

Example 2. Consider the function f(x)=1/x for $x\not=0$. We have

$$\frac{f(a + h) - f(a)}{h} =\frac{\frac{1}{a+h}-\frac{1}{a}}{h}=\frac{a-(a+h)}{h a (a+h)}=\frac{-h}{h a (a+h)}$$

Consequently,

$$\lim_{h \rightarrow 0} \frac{f(a + h) - f(a)}{h} =\lim_{h\rightarrow 0} \frac{-1}{a (a+h)}=-\frac{1}{a^2}.$$

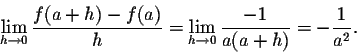
Have you noticed? The algebraic trick in both of the examples above has been to factor out "h" in the numerator, so that we can cancel it with the "h" in the denominator! This is what you try to do whenever you are asked to compute a derivative using the limit definition.

You may believe that every function has a derivative. Unfortunately that is not the case.

Example 3. Let us discuss the derivative of f(x) = |x| at 0. We have

$$\frac{f(0 + h) - f(0)}{h} = \frac{\vert h\vert - 0}{h} = \frac{\vert h\vert}{h}\cdot$$

But

$$\lim_{h \rightarrow 0+} \frac{\vert h\vert}{h} = 1 \;\;\mbox{and}\;\; \lim_{h \rightarrow 0-} \frac{\vert h\vert}{h} = -1$$

which implies that f '(0) does not exist.

Remark. This example is interesting. Even though the derivative at the point does not exist, the right and the left limit of the ratio do exist. In fact, if we use the slope-interpretation of the derivative we see that this means that the graph has two lines close to it at the point under consideration. They could be seen as "half-tangents". See Picture.

So let's push it a little bit more and ask whether a function always has a tangent or half-tangents at any point. That is not the case either.

Example 4. Let us consider the function 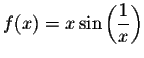$f(x) = x \sin\left(\displaystyle \frac{1}{x}\right)$ for $x \neq 0$, with f(0) = 0. We have

$$\frac{f(0 + h) - f(0)}{h} = \frac{h \sin\left(\displaystyle \frac{1}{h}\right)}{h} = \sin\left(\frac{1}{h}\right)\cdot$$

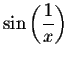Recall that the function $\sin\left(\displaystyle \frac{1}{x}\right)$ has no limit when x goes to 0. So the function has no derivative and no half-derivatives as well at x=0.

What else can go wrong?

Example 5. Consider the function $f(x) = \sqrt{\vert x\vert}$. Then we have

$$\frac{f(0 + h) - f(0)}{h} = \frac{\sqrt{\vert h\vert}}{h} = \frac{1}{\sqrt{\vert h\vert}}\cdot$$

Since

$$\lim_{h \rightarrow 0} \frac{1}{\sqrt{\vert h\vert}} = +\infty$$

then f '(0) does not exist. But observe that the graph as a geometric figure has a tangent -- albeit vertical:

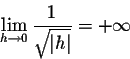
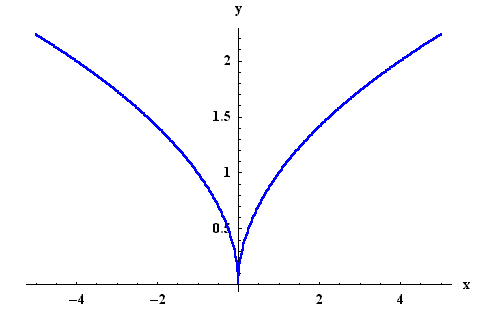In fact, the way the concept of the tangent line was introduced is based on the notion of slope. You already know that vertical lines do not have slopes. So we say that the derivative does not exist whenever the tangent line is vertical. Nevertheless keep in mind that when the limit giving the derivative is $\pm \infty$then the function has a vertical tangent line at the point.

It can be quite laborious (or impossible) to compute the derivative by hand as we have done so far. In the next pages we will show how techniques of differentiation help bypass the limit calculations and make our life much easier.

Exercise 1. Find the derivative of

$$f(x)=\sqrt{x}.$$

Answer.

Exercise 2. Discuss the differentiability of

$$f(x) = \vert x^2 - x\vert\;\cdot$$

Answer.

Exercise 3. We say that the graph of f(x) has a cusp at (a,f(a)), if f(x) is continuous at a and if the following two conditions hold:

1.

$f'(x) \rightarrow +\infty$ as $x \rightarrow a$ from one side (left or right);

2.

$f'(x) \rightarrow -\infty$ as $x \rightarrow a$ from the other side.

Determine whether f(x) = x^4/3 and g(x) = x^3/5 have a cusp at (0,0).

Answer.

Exercise 4. Show that if f '(a) exists, then we have

$$\lim_{h \rightarrow 0} \frac{f(a+h) - f(a-h)}{h} = 2 f'(a)\;.$$

Answer.

Exercise 5. A spherical balloon is being inflated. Find the rate at which its volume V is changing with respect to the radius.

Answer.

[Back] [Next] [Trigonometry] [Calculus] [Geometry] [Algebra] [Differential Equations] [Complex Variables] [Matrix Algebra]

S.O.S MATHematics home page

Copyright © 1999-2004 MathMedics, LLC. All rights reserved.
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA