 Application: A Bouncing Ball
Application: A Bouncing Ball
 Application: A Bouncing Ball
Application: A Bouncing Ball
Suppose you drop a basketball from a height of 10 feet. After it
hits the floor, it reaches a height of
7.5 = 10 . ![]() feet; after it his the floor for the second time, it reaches a
height of
5.625 = 7.5 .
feet; after it his the floor for the second time, it reaches a
height of
5.625 = 7.5 . ![]() = 10 .
= 10 . ![]()
![]()
![]() feet, and so on and so on.
feet, and so on and so on.
Does the ball ever come to rest, and if so, what total vertical distance will it have traveled?
 |
This will lead to summing a geometric series, but let us first investigate, what happens to a ball being dropped from a height h.
Since the ball is subject to free fall, at time t (in seconds) the ball will be at height
Let dn be the distance (in feet) the ball has traveled when it hits the floor for the nth time, and let tn be the time (in seconds) it takes the ball to hit the floor for the nth time.
Clearly d1 = 10. After the ball has hit the floor for the first
time it rises
10 . ![]() feet and then drops the same
distance. Consequently
feet and then drops the same
distance. Consequently
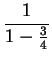 = 70.
= 70.
Let's do the same kind of computations for time: We already computed that
 .
.
 + 2 .
+ 2 .  .
.
 + 2 .
+ 2 .  + 2 .
+ 2 .  + ...
+ ...
 + 2 .
+ 2 . 
 + ...
+ ...  + 2 .
+ 2 .  .
. 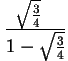
Read this again slowly: Even though the ball bounces infinitely often, it comes to rest after a little more than 11 seconds!
Below you find a table for dn and tn for t = 1,..., 50:
|
|
|
|
| 1 |
|
|
| 2 |
|
|
| 3 |
|
|
| 4 |
|
|
| 5 |
|
|
| 6 |
|
|
| 7 |
|
|
| 8 |
|
|
| 9 |
|
|
| 10 |
|
|
| 11 |
|
|
| 12 |
|
|
| 13 |
|
|
| 14 |
|
|
| 15 |
|
|
| 16 |
|
|
| 17 |
|
|
| 18 |
|
|
| 19 |
|
|
| 20 |
|
|
| 21 |
|
|
| 22 |
|
|
| 23 |
|
|
| 24 |
|
|
| 25 |
|
|
| 26 |
|
|
| 27 |
|
|
| 28 |
|
|
| 29 |
|
|
| 30 |
|
|
| 31 |
|
|
| 32 |
|
|
| 33 |
|
|
| 34 |
|
|
| 35 |
|
|
| 36 |
|
|
| 37 |
|
|
| 38 |
|
|
| 39 |
|
|
| 40 |
|
|
| 41 |
|
|
| 42 |
|
|
| 43 |
|
|
| 44 |
|
|
| 45 |
|
|
| 46 |
|
|
| 47 |
|
|
| 48 |
|
|
| 49 |
|
|
| 50 |
|
|
 S.O.S MATHematics home page
S.O.S MATHematics home page