Improper Integrals: Introduction

Recall that the definition of an integral requires the function f(x) to be bounded on the bounded interval [a,b] (where a and b are two real numbers). It is natural then to wonder what happens to this definition if

1

the function f(x) becomes unbounded (we call this case Type I);

2

the interval [a,b] becomes unbounded (that is or )(we call this case Type II).

In both cases, we say that the integral is improper.

Case Type I: Consider the function f(x) defined on the interval [a,b] (where a and b are real numbers). We have two cases f(x) becomes unbounded around a or unbounded around b (see the images below)

and

For the sake of illustration, we considered a positive function. The integral represents the area of the region bounded by the graph of f(x), the x-axis and the lines x=a and x=b. Assume f(x) is unbounded at a. Then the trick behind evaluating the area is to compute the area of the region bounded by the graph of f(x), the x-axis and the lines x=c and x=b. Then we let c get closer and closer to a (check the figure below)

Hence we have

Note that the integral is well defined. In other words, it is not an improper integral.
If the function is unbounded at b, then we will have

Remark. What happened if the function f(x) is unbounded at more than one point on the interval [a,b]?? Very easy, first you need to study f(x) on [a,b] and find out where the function is unbounded. Let us say that f(x) is unbounded at and for example, with . Then you must choose a number between and (that is ) and then write

Then you must evaluate every single integral to obtain the integral . Note that the single integrals do not present a bad behavior other than at the end points (and not for both of them).

Example. Consider the function defined on [0,1]. It is easy to see that f(x) is unbounded at x = 0 and . Therefore, in order to study the integral

we will write

and then study every single integral alone.

Case Type II: Consider the function f(x) defined on the interval or . In other words, the domain is unbounded not the function (see the figures below).

and

The same as for the Type I, we considered a positive function just for the sake of illustrating what we are doing. The following picture gives a clear idea about what we will do (using the area approach)

So we have

and

Example. Consider the function 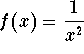defined on . We have

On the other hand, we have

Hence we have

It may happen that the function f(x) may have Type I and Type II behaviors at the same time. For example, the integral

is one of them. As we did before, we must always split the integral into a sum of integrals with one improper behavior (whether Type I or Type II) at the end points. So for example, we have

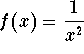The number 1 may be replaced by any number between 0 and since the function has a Type I behavior at 0 only and of course a Type II behavior at .

Convergence and Divergence of improper integrals will be discussed in the next pages.

[Geometry] [Algebra] [Differential Equations] [Calculus] [Complex Variables] [Matrix Algebra] [Trigonometry]

S.O.S MATHematics home page

Copyright © 1999-2004 MathMedics, LLC. All rights reserved.
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA