Some Special Limits

Here we will discuss some important limits that everyone should be aware of. They are very useful in many branches of science.

Example: Show using the Logarithmic function that

,

for any a > 0.

Answer: Set . We have

. ln(a)

Clearly, we have

.

Hence,

which translates into

.

Example: Show that

.

Answer: We will make use of the integral while the Hôpital Rule would have done a cleaner job. We have

so

.

For , we have , which is equivalent to . Hence,

.

But,

.

Therefore, putting the stuff together, we arrive at

.

Since,

,

as n goes to and 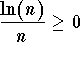, the Pinching Theorem gives

.

The difficulty in this example was that both the numerator and denominator grow when n gets large. But, what this conclusion shows is that n grows more powerfully than .

As a direct application of the above limit, we get the next one:

Example: Show that

.

Answer: Set . We have

.

Clearly, we have (from above)

.

Hence,

,

which translates into

.

The next limit is extremely important and I urge the reader to be aware of it all the time.

Example: Show that

,

for any number a.

Answer: There are many ways to see this. We will choose one that involves a calculus technique. Let us note that it is equivalent to show that

.

Do not worry about the domain of , since for large n, the expression will be a positive number (close to 1). Consider the function

and f(0) = 1. Using the definition of the derivative of , we see that f(x) is continuous at 0, that is, . Hence, for any sequence which converges to 0, we have

.

Now, set

.

Clearly we have . Therefore, we have

.

But, we have

,

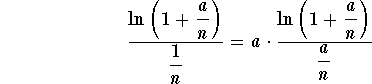which clearly implies

.

Since

,

we get

.

The next example, is interesting because it deals with the new notion of series.

Example: Show that

Answer: There are many ways to handle this sequence. Let us use calculus techniques again. Consider the function

.

We have

and

,

for any . Note that for any , we have

,

hence

,

which gives

.

Since

,

we get

.

In particular, we have

.

Therefore, since , we must have

.

[Trigonometry] [Calculus] [Geometry] [Algebra] [Differential Equations] [Calculus] [Complex Variables] [Matrix Algebra]

S.O.S MATHematics home page

Copyright © 1999-2004 MathMedics, LLC. All rights reserved.
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA