Differential Equations

Differential Equations Practice Exams

Time: 1 hour

Problem 1. Find the solution to:

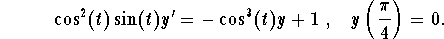
Problem 2. Solve the initial value problem

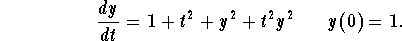
Problem 3. The fox squirrel is a small mammal native to the Rocky Mountains. These squirrels are very territorial:

if the population is large, their rate of growth decreases or even becomes negative;
if the population is too small, fertile adults run the risk of not being able to find suitable mates, so again the rate of growth is negative

The carrying capacity N indicates what population is too big, and the sparsity parameter M indicates when the population is too small. A mathematical model which will agree with the above assumptions is the modified logistic model:

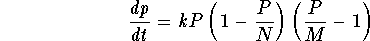
1.: Find the equilibrium (critical) points. Classify them as : source, sink or node. Justify your answers.
2.: Sketch the slope-field.
3.: Assume N=100 and M=1 and k = 1. Sketch the graph of the solution which satisfies the initial condition y(0)=20.
4.: Assume that squirrels are emigrating (from a certain region) with a fixed rate E. Write down the new differential equation.
Discuss the equilibrium (critical) points under the parameter E. When do you observe a bifurcation?

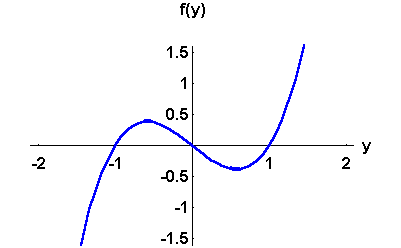
Problem 4. Consider the autonomous differential equation 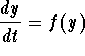tex2html_wrap_inline50 where the graph of f(y) is

1.

Sketch the Slope Fields of this differential equation
Hint: the graph of the solutions and the graph of f(y) are two different entities!

2.

Sketch the graph of the solution to the IVP

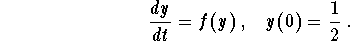
Find the

3.

Sketch the graph of the solution to the IVP

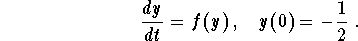
Find the

If you would like to check your answers, click on Answer.