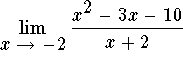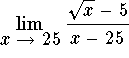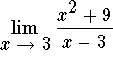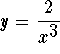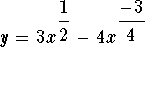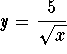Math for Social Sciences II. Practice Exam.

Find the limit of the following functions: (12 points)
Find the feasibility region in the following. Label any ``corner'' points of the region. (16 pts)
, , , .
Minimize z=4x+12y subject to: (18 pts)
, , , .
Find the derivative of each of the following: (20 pts)
Use the definition of the derivative to find f'(3) if f(x)=2x+4 (7pts)
The cost of producing x pizzas is (7 pts)
- Find the marginal cost C'(x)
- Find and interpret C'(500)
- Find the exact cost to produce the 501st pizza.
- How are the answers in b and c related?
Set up the following problems. Indicate what the variables represent, the objective function and any constraints. (10 each)
- A candy company has 300 kilograms of chocolate-covered nuts and 100 kilograms of chocolate covered raisins to be sold as two different mixes. One mix will contain 1/2 nuts and 1/2 raisins and will sell for $4 per kilogram. The other mix will contain 3/4 nuts and 1/4 raisins and will sell for $6.50 per kilogram. How many kilograms of each mix should the company prepare for maximum revenue?
- A bakery makes both cakes and cookies. Each batch of cakes requires 2 hours in the oven and 3 hours in the decorating room. Each batch of cookies needs 1 and a half hours in the oven and 2/3 of an hour in the decorating room. The oven is available no more than 15 hours a day while the decorating room can be used no more than 13 hours a day. How many batches of cakes and cookies should the bakery make in order to maximize profits if cookies produce a profit of $20 per batch and cakes produce a profit of $30 per batch?

If you would like to check your answers, click on Answer.

If you would like to go back to the original menu, click on Menu.

Tue Nov 07 21:45:49 MDT 1997