 First Order Linear Equations
First Order Linear Equations

A first order linear differential equation has the
following form:
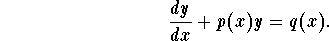
The general solution is given by
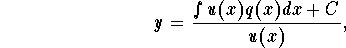
where
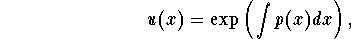
called the integrating factor. If an initial condition is given, use it to find the constant C.
Here are some practical steps to follow:
- 1.
- If the differential equation is given as
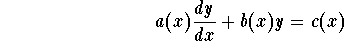 ,
,
rewrite it in the form
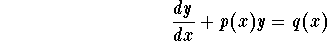 ,
,
where
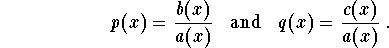
- 2.
- Find the integrating factor
 .
.
- 3.
- Evaluate the integral

- 4.
- Write down the general solution
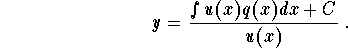 .
.
- 5.
- If you are given an IVP, use the initial condition to find
the constant C.
Example: Find the particular solution of:
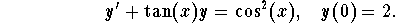
Solution: Let us use the steps:
-
- Step 1: There is no need for rewriting the differential
equation. We have
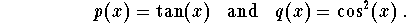
-
- Step 2: Integrating factor
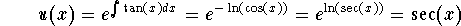 .
.
-
- Step 3: We have
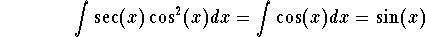 .
.
-
- Step 4: The general solution is given by
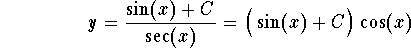 .
.
-
- Step 5: In order to find the particular solution to the given
IVP, we use the initial condition to find C. Indeed, we have
 .
.
Therefore the solution is
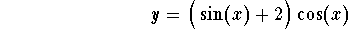 .
.
Note that you may not have to do the last step if you are asked to
find the general solution (not an IVP).
If you would like more practice, click on
Example.

[Differential Equations]
[Separable Equations]
[Next Example]
[Geometry]
[Algebra]
[Trigonometry ]
[Calculus]
[Complex Variables]
[Matrix Algebra]
 S.O.S MATHematics home page
S.O.S MATHematics home page

Copyright © 1999-2004 MathMedics, LLC. All rights reserved.
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA
 First Order Linear Equations
First Order Linear Equations  First Order Linear Equations
First Order Linear Equations 
![]()
![]()
![]()
![]() ,
, ![]() ,
, ![]()
![]() .
. ![]() .
. ![]()
![]()
![]() .
. ![]() .
. ![]() .
. ![]() .
. ![]() .
. 
 S.O.S MATHematics home page
S.O.S MATHematics home page 