Hyperbolic Functions

The hyperbolic functions enjoy properties similar to the trigonometric functions; their definitions, though, are much more straightforward:

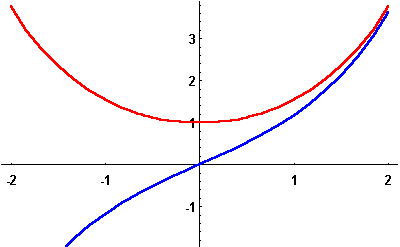
Here are their graphs: the (pronounce: "kosh") is pictured in red, the function (rhymes with the "Grinch") is depicted in blue.

As their trigonometric counterparts, the function is even, while the function is odd.

Their most important property is their version of the Pythagorean Theorem.

• 

The verification is straightforward:

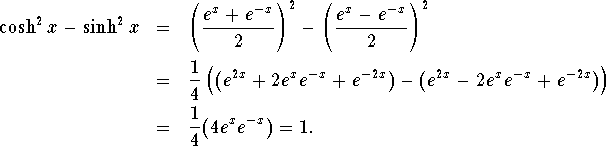
While , , parametrizes the unit circle, the hyperbolic functions , , parametrize the standard hyperbola , x>1.

In the picture below, the standard hyperbola is depicted in red, while the point for various values of the parameter t is pictured in blue.

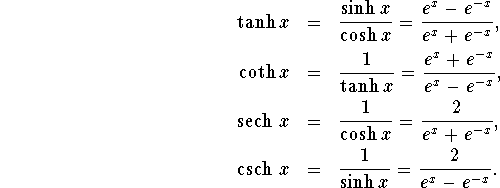
The other hyperbolic functions are defined the same way, the rest of the trigonometric functions is defined:

tanh x	coth x
sech x	csch x

For every formula for the trigonometric functions, there is a similar (not necessary identical) formula for the hyperbolic functions:

Let's consider for example the addition formula for the hyperbolic cosine function:

• 

Start with the right side and multiply out:

Try it yourself!

Prove the addition formula for the hyperbolic sine function:

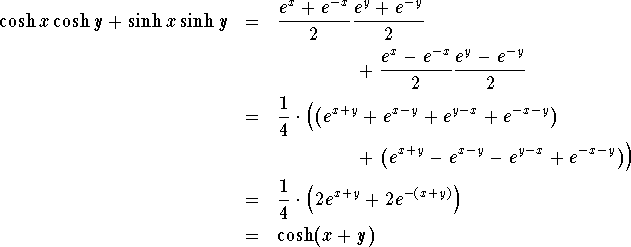
Show that .

Here is the answer.

Click here to go to the inverse hyperbolic functions.

Copyright © 1999-2004 MathMedics, LLC. All rights reserved.
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA