RULES OF LOGARITHMS - Example

Let a be a positive number such that a does not equal 1, let n be a real number, and let u and v be positive real numbers.

Logarithmic Rule 2:

Example 4: Expand 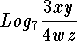and write the answer in terms of base e.

Solution: Write 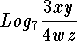as using Rule 2.
Now expand further by using Rule 1.

Now convert the above terms to the base e.

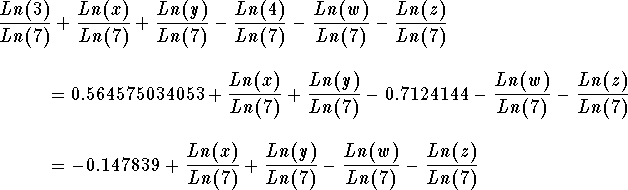
as long as x > 0, y > 0, z > 0, and w > 0.
Since

the two expressions are equivalent. This means that if you substitute the same values of x, y, w, and z in both expressions, the results will be equal.

Let's check the answer by letting x = 2, y = 3, w = 4, and z =5. Substituting these values in the original problem yields

Now let's substitute the same values in the final expression.

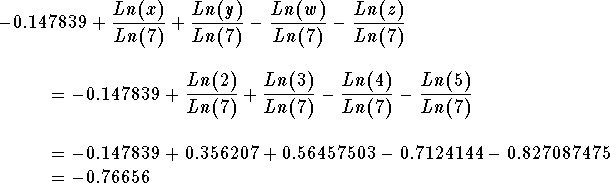
Since the check works, you have successfully worked this problem.

If you would like to review another example, click on Example.

S.O.S. MATHematics home page