 SOLVING LOGARITHMIC EQUATIONS
SOLVING LOGARITHMIC EQUATIONS SOLVING LOGARITHMIC EQUATIONS
SOLVING LOGARITHMIC EQUATIONS
Note:
If you would like an in-depth review of logarithms, the rules of logarithms, logarithmic functions and logarithmic equations, click on logarithmic functions.
Solve for x in the following equation.
Problem 8.4d:
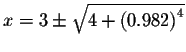 and
and
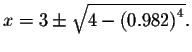 The
approximate answers are
The
approximate answers are
![]() 1.2478358458,
4.75216415412, and
1.2478358458,
4.75216415412, and
![]()
Solution:
The above equation is valid only if the expression
![]() The expression
The expression
![]() is valid when
is valid when ![]() and
and ![]() Another way of saying this is that the
domain is the set of real numbers where
Another way of saying this is that the
domain is the set of real numbers where
![]()
Note: If, when solving the above problem, you simplify the logarithmic
equation
![]() to
to
![]() you will lose half of your solutions.
Why?
you will lose half of your solutions.
Why?
Recall that
![]() is valid for all
real numbers that are not equal to 1 or 5. However,
is valid for all
real numbers that are not equal to 1 or 5. However,
![]() is valid for the set of real numbers
is valid for the set of real numbers
![]() This means that
This means that
![]() only over the set of real numbers
only over the set of real numbers
![]()
So be careful when you simplify a logarithmic equation before solving it.
Convert the logarithmic equation
![]() to an equivalent exponential equation with base 0.982.
to an equivalent exponential equation with base 0.982.
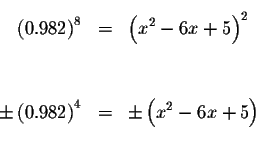
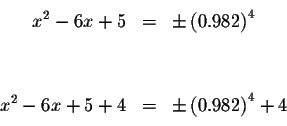
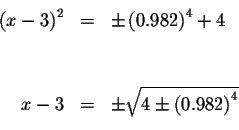
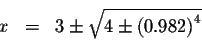
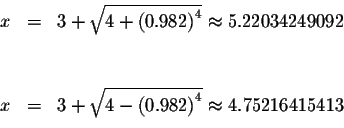
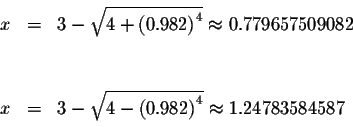
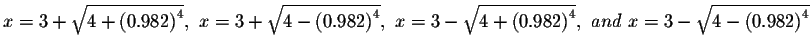 .
The approximate answers are
.
The approximate answers are
These answers may or may not be the solutions. You must check them
numerically or graphically.
Numerical Check:
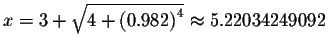 by substituting
5.22034249092 in the original equation for
x. If the left side of the equation equals the right side of the equation
after the substitution, you have found the correct answer.
by substituting
5.22034249092 in the original equation for
x. If the left side of the equation equals the right side of the equation
after the substitution, you have found the correct answer.
Left Side:
![]()

Right Side:![]()
Since the left side of the original equation is equal to the right side of
the original equation after we substitute the value
5.22034249092 for x,
then
![]() is a solution.
is a solution.
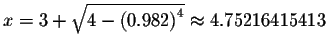 by substituting
4.75216415413 in the original equation for
x. If the left side of the equation equals the right side of the equation
after the substitution, you have found the correct answer.
by substituting
4.75216415413 in the original equation for
x. If the left side of the equation equals the right side of the equation
after the substitution, you have found the correct answer.
Left Side:
![]()

Right Side:![]()
Since the left side of the original equation is equal to the right side of
the original equation after we substitute the value
4.75216415413 for x,
then
![]() is a solution.
is a solution.
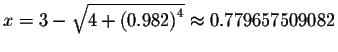 by substituting
0.779657509082 in the original equation
for x. If the left side of the equation equals the right side of the
equation after the substitution, you have found the correct answer.
by substituting
0.779657509082 in the original equation
for x. If the left side of the equation equals the right side of the
equation after the substitution, you have found the correct answer.
Left Side:
![]()

Right Side:![]()
Since the left side of the original equation is equal to the right side of
the original equation after we substitute the value
0.779657509082 for x,
then
![]() is a solution.
is a solution.
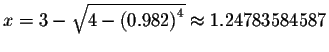 by substituting
1.24783584587 in the original equation for
x. If the left side of the equation equals the right side of the equation
after the substitution, you have found the correct answer.
by substituting
1.24783584587 in the original equation for
x. If the left side of the equation equals the right side of the equation
after the substitution, you have found the correct answer.
Left Side:
![]()

Right Side:![]()
Since the left side of the original equation is equal to the right side of
the original equation after we substitute the value
1.24783584587 for x,
then
![]() is a solution.
is a solution.
Graphical Check:
You can also check your answer by graphing
![]() (formed by subtracting the right side of the
original equation from the left side). Look to see where the graph crosses
the x-axis; that will be the real solution. Note that the graph crosses the
x-axis at four places:
(formed by subtracting the right side of the
original equation from the left side). Look to see where the graph crosses
the x-axis; that will be the real solution. Note that the graph crosses the
x-axis at four places:
![]() ,
and
5.22034249092. This means that
,
and
5.22034249092. This means that
![]() ,
and
5.22034249092 are the real solutions.
,
and
5.22034249092 are the real solutions.
If you have trouble graphing the function
![]() ,
graph the equivalent function
,
graph the equivalent function
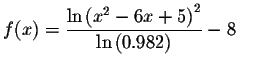 .
.
If you would like to go to the next section, click on next.
If you would like to go back to the previous section, click on
previous.
If you would like to go back to the equation table of contents, click on
contents.
This site was built to accommodate the needs of students. The topics and problems are what students ask for. We ask students to help in the editing so that future viewers will access a cleaner site. If you feel that some of the material in this section is ambiguous or needs more clarification, or you find a mistake, please let us know by e-mail.

