 SOLVING LOGARITHMIC EQUATIONS
SOLVING LOGARITHMIC EQUATIONS SOLVING LOGARITHMIC EQUATIONS
SOLVING LOGARITHMIC EQUATIONS
Note:
If you would like an in-depth review of logarithms, the rules of logarithms, logarithmic functions and logarithmic equations, click on logarithmic function.
Solve for x in the following equation.
Example 2:
The equation is valid only if
x2-7x+2>0 or
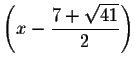
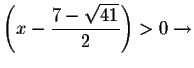
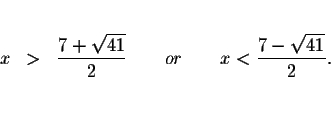
Isolate the log term..
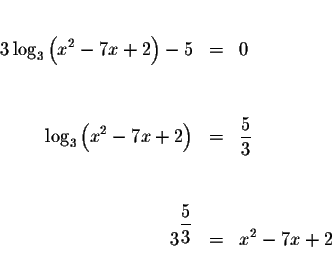
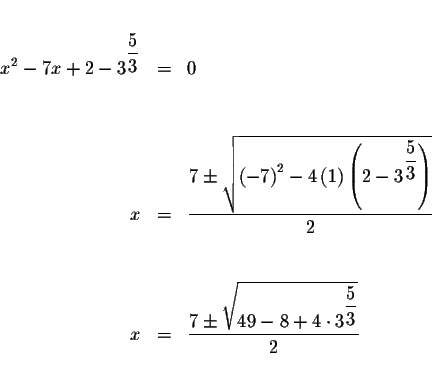
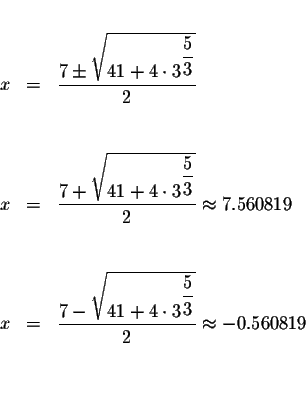
The exact answers are
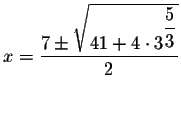 and the approximate answers are 7.560819 and
-0.560819.
and the approximate answers are 7.560819 and
-0.560819.
These answers may or may not be the solutions. You must check them with the original equation, either by a numerical substitution or by graphing.
Numerical Check:
Check the answer
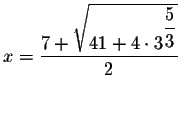 by
substituting 7.560819 in the original equation for x. If the left side of
the equation equals the right side of the equation after the substitution,
you have found the correct answer.
by
substituting 7.560819 in the original equation for x. If the left side of
the equation equals the right side of the equation after the substitution,
you have found the correct answer.
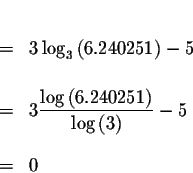
Check the answer
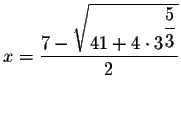 by
substituting -0.560819 in the original equation for x. If the left side of
the equation equals the right side of the equation after the substitution,
you have found the correct answer.
by
substituting -0.560819 in the original equation for x. If the left side of
the equation equals the right side of the equation after the substitution,
you have found the correct answer.
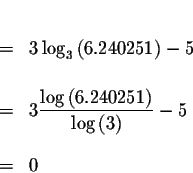
Graphical Method:
Graph the function
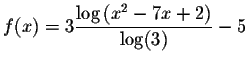
If you would like to work another example, click on example.
If you would like to test yourself by working some problems similar to this
example, click on problem.
If you would like to go back to the previous section, click on previous.
If you would like to go back to the equation table of contents, click on
contents.
This site was built to accommodate the needs of students. The topics and problems are what students ask for. We ask students to help in the editing so that future viewers will access a cleaner site. If you feel that some of the material in this section is ambiguous or needs more clarification, or you find a mistake, please let us know by e-mail.

