
 SOLVING TRIGONOMETRIC EQUATIONS
SOLVING TRIGONOMETRIC EQUATIONS

Note: If you would like a review of trigonometry, click on trigonometry.
Example 2: Solve for x in the following equation.
There are an infinite number of solutions to this problem.
First isolate the cosine term.
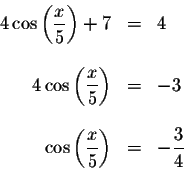
To solve for x, we have to isolate x. How do we isolate the x? We could take the inverse (arccosine) of both sides. However, inverse functions can only be applied to one-to-one functions and the cosine function is not one-to-one.
Let's restrict the domain so the function is one-to-one on the restricted
domain while preserving the original range. The tangent function is
one-to-one on the interval
![]() If we restrict the
domain of the cosine function to that interval , we can take the arccosine
of both sides of the equation and isolate the x.
If we restrict the
domain of the cosine function to that interval , we can take the arccosine
of both sides of the equation and isolate the x.
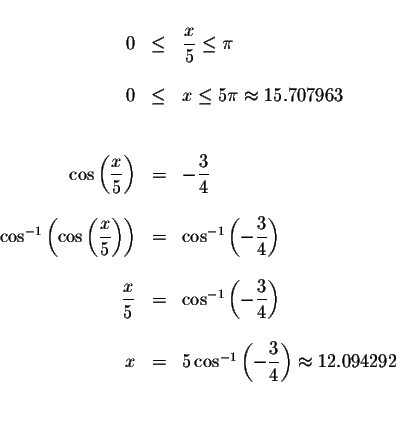
The angle x is the reference angle. We know that
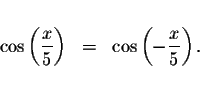
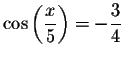 ,
then
,
then
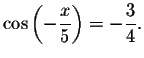
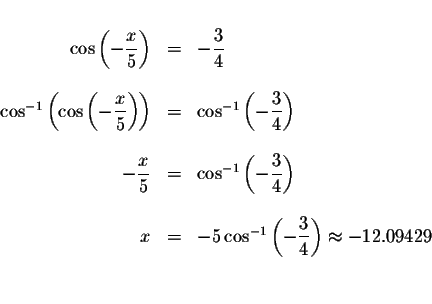
The period of ![]() equals
equals ![]() and the period of
and the period of
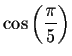 equals
equals ![]() ,
this means other solutions exists
every
,
this means other solutions exists
every ![]() units. The exact solutions are
units. The exact solutions are

where n is an integer.
The approximate values of these solutions are
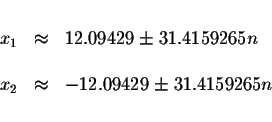
where n is an integer.
You can check each solution algebraically by substituting each solution in the original equation. If, after the substitution, the left side of the original equation equals the right side of the original equation, the solution is valid.
You can also check the solutions graphically by graphing the function formed by subtracting the right side of the original equation from the left side of the original equation. The solutions of the original equation are the x-intercepts of this graph.
Algebraic Check:
Check solution x=12.09429
Left Side:
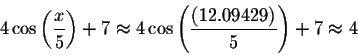
Right Side: ![]()
Since the left side of the original equation equals the right side of the original equation when you substitute 12.09429 for x, then 12.09429 is a solution.
Check solution x=-12.09429
Left Side:
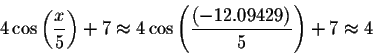
Right Side: ![]()
Since the left side of the original equation equals the right side of the original equation when you substitute -12.09429 for x, then -12.09429 is a solution.
We have just verified algebraically that the exact solutions are
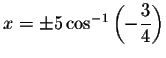 and these solutions repeat every
and these solutions repeat every
![]() units. The approximate values of these solutions are
units. The approximate values of these solutions are
![]() and these solutions repeat every
and these solutions repeat every
![]() units.
units.
Graphical Check:
Graph the equation
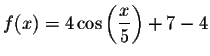 (formed by
subtracting the right side of the original equation from the left side of
the original equation). Note that the graph crosses the x-axis many times
indicating many solutions. Let's check a few of these x-intercepts against
the solutions we derived.
(formed by
subtracting the right side of the original equation from the left side of
the original equation). Note that the graph crosses the x-axis many times
indicating many solutions. Let's check a few of these x-intercepts against
the solutions we derived.
Verify the graph crosses the x-axis at 12.09429. Since the period is
![]() ,
you can verify that the graph also crosses the
x-axis again at
,
you can verify that the graph also crosses the
x-axis again at
![]() and at
and at
![]() etc.
etc.
Verify the graph crosses the x-axis at
-12.09429. Since the period is
![]() ,
you can verify that the graph also crosses the
x-axis again at
,
you can verify that the graph also crosses the
x-axis again at
![]() and at
and at
![]() etc.
etc.
Note: If the problem were to find the solutions in the interval
![]() ,
then you choose those solutions from the set of infinite
solutions that belong to the set
,
then you choose those solutions from the set of infinite
solutions that belong to the set
![]() In this case,
although there are an infinite number of solutions, none of the solutions
are located in the interval
In this case,
although there are an infinite number of solutions, none of the solutions
are located in the interval
![]()
If you would like to test yourself by working some problems similar to this example, click on Problem.
If you would like to go to the next section, click on Next.
If you would like to go back to the equation table of contents, click on Contents.

