Problems on Improper Integrals

Exercise 1. Decide on the convergence or divergence of

Answer. First notice that the denominator is equal to 0 when x=1. Then the function inside the integral sign is unbounded at x=1. Hence we have two bad points 1 and . So we must split the integral and write

Let us first take care of the integral

We have

Since (by Bertrand's test) the improper integral

is convergent, then by the comparison test the improper integral

Next we take care of the integral

First notice that when , then

One may check this by showing that

On the other hand (and this is the crucial step in this exercise) is to find a polynomial approximation of when . This will be done via Taylor polynomials. Indeed, we have

when , which gives

Hence we have

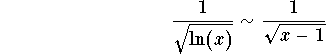
which implies

when . The p-test implies that the improper integral

is convergent. Therefore the limit test implies that the improper integral

is convergent. Putting the two integrals together, we conclude that the improper integral

is convergent.

Exercise 2. Decide on the convergence or divergence of

Answer. The term is never equal for . So let us focus on the term . According to the domain of the tangent function, the only bad points we have to worry about is . Clearly we have

Hence we have an improper integral or Type I at the bad point . Clearly we have

when . So let us approximate when . Again we will use Taylor polynomials, we have

when . This gives

when . Putting the stuff together we get

The p-test implies that the improper integral

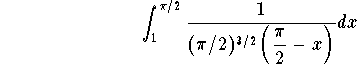
is divergent. Therefore the improper integral

is divergent.

Exercise 3. Decide on the convergence or divergence of

Answer. The only improper behavior is around . Hence this integral is of Type II not of Type I. Therefore no need for splitting it. Note that when , then . Hence

when . The p-test implies that the improper integral

is convergent. Therefore the limit test implies that the improper integral

is convergent.

Problems

Next you will find some not so easy problems on improper integrals. We invite you to solve them and submit the answer to SOS MATHematics. We will publish your answer with your name. Good luck.

Problem 1. First decide on the convergence and divergence of

Then evaluate f(x).

Problem 2. Assume that is continuous. Find , where

Problem 3. Consider the function

Find f(x).

Problem 4. Evaluate

Problem 5. In this problem, we will evaluate

1

Evaluate

2

Let which function (that is f is differentiable and f' is continuous). Show that

3

Evaluate

Problem 6. Decide on convergence or divergence of

where x > 0 and is any real number.

Problem 7. Decide on convergence or divergence of

Problem 8. Decide on convergence or divergence of

If I is convergent, evaluate it.

Problem 9. Find

[Geometry] [Algebra] [Differential Equations] [Calculus] [Complex Variables] [Matrix Algebra] [Trigonometry]

S.O.S MATHematics home page

Copyright © 1999-2004 MathMedics, LLC. All rights reserved.
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA