Integrating Powers and Product of Sines and Cosines
Integrating Powers and Product of Sines and Cosines
These are integrals of the following form:
We have two cases: both m and n are even or at least one of them is
odd.
Suppose n is odd. Hence n = 2k +
1. So 
hold. Therefore, we have
which suggests the substitution 
. Indeed, we have 
and hence
The latest integral is a polynomial function of u which is easy to
integrate.
Remark. Note that if m is odd, then we
will split 
and carry the same calculations. In this case,
the substitution will be 
.
The main idea behind is to use the trigonometric identities
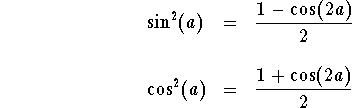
Remark. The following two formulas may be helpful in integrating powers of sine and cosine.
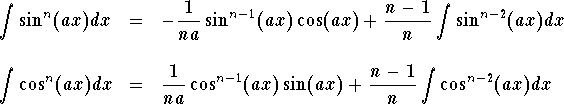
S.O.S MATHematics home page 