Absolute Value Inequalities

Find the solutions of the inequality

|2x-5|>x+1.

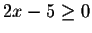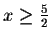First we consider the case where $2x-5\geq 0$ , i.e. $x\geq\frac{5}{2}$ . In this case |2x-5|=2x-5, so we can write the inequality as

2x-5>x+1.

Subtracting x on both sides and adding 5, we get x>6.

Now consider the case 2x-5< 0, i.e. 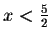$x<\frac{5}{2}$ . In this case |2x-5|=-(2x-5)=5-2x, so we can write the inequality as

5-2x>x+1.

Adding 2x and subtracting 1 on both sides yields

4>3x,

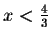so we obtain the requirement that $x<\frac{4}{3}$ .

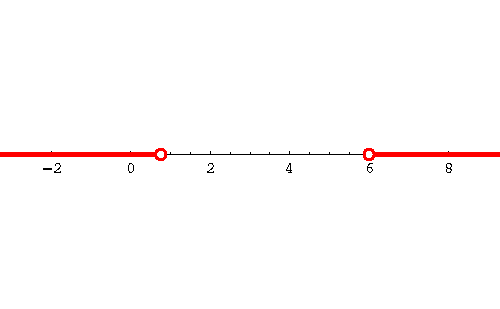
So a real number x is a solution of the original inequality if

$\begin{displaymath}x\geq\frac{5}{2} \mbox{ and } x >6,\end{displaymath}$

or if

$\begin{displaymath}x<\frac{5}{2} \mbox{ and } x<\frac{4}{3}.\end{displaymath}$

Thus the set of solutions is

$\begin{displaymath}(-\infty,\frac{4}{3})\cup(6,\infty).\end{displaymath}$

1998-06-08