RULES OF LOGARITHMS
RULES OF LOGARITHMS
Let a be a positive number such that a does not equal 1, let
n be a real number, and let u and v be positive real numbers.
Logarithmic Rule 1: 
Logarithmic Rule 2:
Logarithmic Rule 3:
Since logarithms are nothing more than exponents, these rules
come from the rules of exponents. Let a be greater than 0 and
not equal to 1, and let n and m be real numbers.
Exponential Rule 1: 
Example: Let a = 5, n = 2, and m = 6.
and
Exponential Rule 2: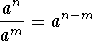 
Example: Let a = 5, n = 2, and m = 6.
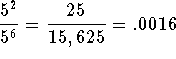and
Exponential Rule 3: 
Example: Let a = 5, n = 2, and m = 6.
and
If you want to review exponential rules in detail with examples and problems, click on Exponential Rules.
If you want to review logarithmic rules in detail with examples
and problems, click on one of the following rules.
S.O.S MATHematics home page