SOLVING EXPONENTIAL EQUATIONS

Note:

• To solve an exponential equation, isolate the exponential term, take the logarithm of both sides and solve.

If you would like an in-depth review of exponents, the rules of exponents, exponential functions and exponential equations, click on exponential function.

Solve for the real number x in the following equation.

Problem 7.7b:

Answer: No Real Solution

Solution:

The first step is to isolate .

Add 20 to both sides of the equation.

Divide both sides of the equation by 5.

The next step is to isolate the variable x.

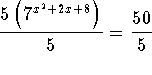
Take the natural logarithm of both sides of the equation..

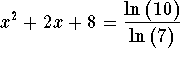
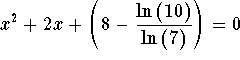
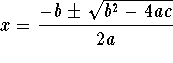Use the Quadratic Formula where a=1, b=2,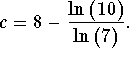

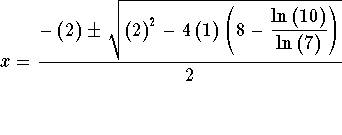
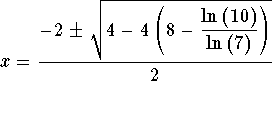
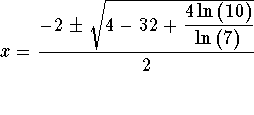
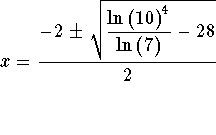
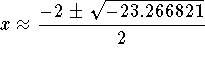
No Real Solution

No Solution.

You can also check your answer by graphing (formed by subtracting the right side of riginal equation from the left side). Look to see where the graph crosses the x-axis; that will be the real solution. Note that the graph never crosses the x-axis. This means that there are no real solutions.

If you would like to review the solution to problem 7.6e, click on Problem

If you would like to go back to the beginning of this section, click on Beginning

If you would like to go back to the equation table of contents, click on Contents

This site was built to accommodate the needs of students. The topics and problems are what students ask for. We ask students to help in the editing so that future viewers will access a cleaner site. If you feel that some of the material in this section is ambiguous or needs more clarification, please let us know by e-mail.

[Algebra] [Trigonometry] [Geometry] [Differential Equations] [Calculus] [Complex Variables] [Matrix Algebra]

S.O.S. MATHematics home page

Copyright © 1999-2004 MathMedics, LLC. All rights reserved.
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA