 The Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus
This theorem bridges the antiderivative concept with the area
problem. Indeed, let f (x) be a function defined and continuous
on [a, b]. Consider the function
F(
x) =
 f
f (
t)
dt
defined on [a, b]. Then we have
Since
 dt = h, we get
dt = h, we get
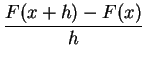
-
f (
x) =


 f
f (
t) -
f (
x)
 dt
dt .
Since F(t) is continuous, we can easily prove that

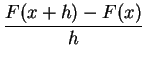
-
f (
x) = 0 .
This means that the function F(x) is differentiable and
F '(x) = f (x). In other words, the function F(x) is an
antiderivative of f (x). From this and what we learned about
antiderivatives, we obtain the following fundamental result:
The Fundamental Theorem of Calculus Let f (x) be
continuous on [a, b]. If F(x) is any antiderivative of
f (x), then
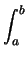 f
f (
x)
dx =
F(
b) -
F(
a) .
Remark. The number
F(b) - F(a) is also denoted by
[F(x)]ba (or
 F(x)
F(x)  ). So
). So
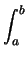 f
f (
x)
dx = [
F(
x)]
ba
Example. We have

cos(
t)
dt = [sin(
t)]
0x = sin(
x) - sin(0) = sin(
x) .
So
Example. We have

 dt
dt = [arctan(
t)]
0x = arctan(
x) .
In particular, we have
Combining the Chain Rule with the Fundamental Theorem of Calculus,
we can generate some nice results. Indeed, let f (x) be
continuous on [a, b] and u(x) be differentiable on [a, b].
Define the function
F(
x) =
 f
f (
t)
dt .
Then the Chain Rule implies that F(x) is differentiable and
F '(
x) =
f u
u(
x)
 u
u '(
x) .
We can generalize this a little bit more to find the derivative
of a function of the form
H(
x) =
 f
f (
t)
dt
where u(x) and v(x) are both differentiable on [a, b]. We
have
H '(
x) =
f v
v(
x)
 v
v '(
x) -
f u
u(
x)
 u
u '(
x) .
Example. Find the derivative of

cos(
t2)
dt .
Set
F(
x) =

cos(
t2)
dt .
From the Fundamental Theorem of Calculus, we know that F(x) is
an antiderivative of cos(x2). We have

cos(
t2)
dt =
F(
x2) .
The Chain Rule then implies that


cos(
t2)
dt
=
F '(
x2)2
x = 2
x cos

(
x2)
2
= 2
x cos(
x4) .
Note that F(x) does not have an explicit form. So it is quite
amazing that even if F(x) is defined via some theoretical
result, we are still able to find the derivative of the given
function.
Example. Find the derivative of
 e-t2dt
e-t2dt .
Set
F(
x) =
 e-t2dt
e-t2dt .
From the Fundamental Theorem of Calculus, we know that F(x) is
an antiderivative of e-x2. We have
 e-t2dt
e-t2dt =
F(
x2) -
F(
x) .
The Chain Rule implies


 e-t2dt
e-t2dt
=
F '(
x2)2
x -
F '(
x) = 2
xe-x4 -
ex2 .
Exercise 1. Let f (x) be a function defined and continuous
on [a, b]. Compare
Answer.
Exercise 2. Find a function F(x) such that
F '(x) = sin(x2) and F(1) = 2.
Answer.
[Back]
[Next]
[Trigonometry]
[Calculus]
[Geometry]
[Algebra]
[Differential Equations]
[Complex Variables]
[Matrix Algebra]
 S.O.S MATHematics home page
S.O.S MATHematics home page
Copyright © 1999-2004 MathMedics, LLC. All rights reserved.
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA
 The Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus
 The Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus
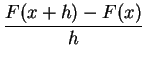 =
= 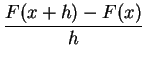 - f (x) =
- f (x) = 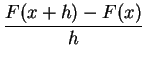 - f (x) = 0 .
- f (x) = 0 .
![]() F(x)
F(x) ![]() ). So
). So
 dt = [arctan(t)]0x = arctan(x) .
dt = [arctan(t)]0x = arctan(x) .
 dt = arctan(1) =
dt = arctan(1) = ![]()
![]()
![]() f (t)dt
f (t)dt![]() and
and ![]()
![]()
![]() f (t)
f (t)![]() dt .
dt .
 S.O.S MATHematics home page
S.O.S MATHematics home page