Mean Value Theorems for Integrals

Let f (x) be continuous on [a, b]. Set

F(x) = $$\int_{a}^{x}$$f (t)dt  .

The Fundamental Theorem of Calculus implies F '(x) = f (x). The Mean Value Theorem implies the existence of c $\in$ (a, b) such that

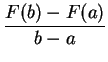$${\frac{F(b) - F(a)}{b-a}}$$ = F '(c),    or equivalently    F(b) - F(a) = F '(c)$$\Big($$b - a$$\Big)$$

which implies

$$\int_{a}^{b}$$f (t)dt = f (c)$$\Big($$b - a$$\Big)$$  .

This is known as the First Mean Value Theorem for Integrals. The point f (c) is called the average value of f (x) on [a, b].

As the name "First Mean Value Theorem" seems to imply, there is also a Second Mean Value Theorem for Integrals:

Second Mean Value Theorem for Integrals. Let f (x) and g(x) be continuous on [a, b]. Assume that g(x) is positive, i.e. g(x) $\geq$ 0 for any x $\in$ [a, b]. Then there exists c $\in$ (a, b) such that

$$\int_{a}^{b}$$f (t)g(t)dt = f (c)$$\int_{a}^{b}$$g(t)dt  .

The number f (c) is called the g(x)-weighted average of f (x) on the interval [a, b].

As an application one may define the Center of Mass of one-dimensional non-homogeneous objects such as a metal rod. If the object is homogeneous and lying on the x-axis from x = a to x = b, then its center of mass is simply the midpoint

$${\frac{a+b}{2}}$$   ^.

If, on the other hand, the object is not homogeneous with $\lambda$(x) being the density function, then the total mass M is

M = $$\int_{a}^{b}$$$$\lambda$$(x)dx  .

The density-weighted average x_c is defined by

$$\int_{a}^{b}$$x$$\lambda$$(x)dx = x_c$$\int_{a}^{b}$$$$\lambda$$(x)dx = x_cM,

or equivalently

x_c = $${\frac{1}{M}}$$$$\int_{a}^{b}$$x$$\lambda$$(x)dx  .

The point x_c is the center of mass of the object.

Example. A rod of length L is placed on the x-axis from x = 0 to x = L. Assume that the density $\lambda$(x) of the rod is proportional to the distance from the x = 0 endpoint of the rod. Let us find the total mass M and the center of mass x_c of the rod. We have $\lambda$(x) = kx, for some constant k > 0. We have

M = $$\int_{0}^{L}$$$$\lambda$$(x)dx = $$\int_{0}^{L}$$k x dx = k$${\frac{L^2}{2}}$$ = $${\frac{kL^2}{2}}$$

and

x_c = $${\frac{1}{M}}$$$$\int_{0}^{L}$$x$$\lambda$$(x)dx = $${\frac{1}{M}}$$$$\int_{0}^{L}$$kx²dx = $${\frac{1}{M}}$$k$${\frac{L^3}{3}}$$ = $${\textstyle\frac{2}{3}}$$L  .

If the rod was homogeneous, then the center of mass would be at the midpoint of the rod. Now it is closer to the endpoint x = L. This is not surprising since there is more mass at this end.

Exercise 1. Find the average value of

f (x) = sec²(x)

for x $\in$ [0,$\pi$/4].

Answer.

Exercise 2. Find the average value of

f (x) = $$\sqrt{x}$$

for x $\in$ [0, 4].

Answer.

Exercise 3. Assume that f (x) be continuous and increasing on [a, b]. Compare

f (a)(b - a)    and    $$\int_{a}^{b}$$f (x)dx  .

Answer.

[Back] [Trigonometry] [Calculus] [Geometry] [Algebra] [Differential Equations] [Complex Variables] [Matrix Algebra]

S.O.S MATHematics home page

Copyright © 1999-2004 MathMedics, LLC. All rights reserved.
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA