 Continuity
Continuity
 Continuity
Continuity
We have seen that any polynomial function P(x) satisfies:
Definition. Let f(x) be a function defined on an interval
around a. We say that f(x) is continuous at a iff
Note that the continuity of f(x) at a means two things:
Basic properties of limits imply the following:
Theorem. If f(x) and g(x) are continuous at a. Then
Remark. Many functions are not defined on open intervals.
In this case, we can talk about one-sided continuity. Indeed,
f(x) is said to be continuous from the left at a iff
Example. The function
![]() is defined for
is defined for ![]() .
So we can not talk about left-continuity of f(x) at
0. But since
.
So we can not talk about left-continuity of f(x) at
0. But since
This concept is also important for step-functions.
Example. Consider the function
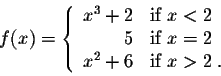
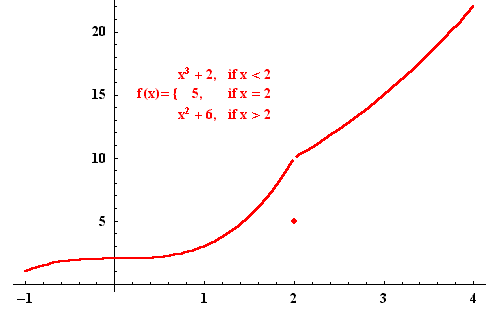 |
Exercise 1. Find A which makes the function
Definition. For a function f(x) defined on a set S, we
say that f(x) is continuous on S iff f(x) is continuous for
all
Example. We have seen that polynomial functions are
continuous on the entire set of real numbers. The same result
holds for the trigonometric functions
The following two exercises discuss a type of functions hard to
visualize. But still one can study their continuity properties.
Exercise 2. Discuss the continuity of
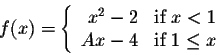
continuous at x=1.
![]() .
.
![]() and
and ![]() .
.
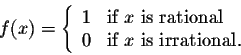
Exercise 3. Let us modify the previous function: Discuss the
continuity of
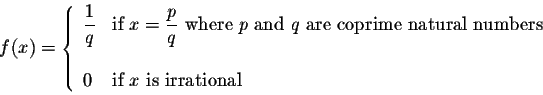
for
![]() .
(Two natural numbers p and q are coprime,
if their greatest common divisor equals 1.)
.
(Two natural numbers p and q are coprime,
if their greatest common divisor equals 1.)
 S.O.S MATHematics home page
S.O.S MATHematics home page
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA